A classification a bit strange

This is the text of the poster you can see here.

Where Polygons are Placed

It is not difficult in Mathematics (and in particular in some areas) to come across some apparently “harmless” questions, which are easy to formulate and tell, but with a really complicated solution. Some of these questions (for example, the 4-colour theorem or the Fermat’s theorem) are famous for laypeople too. Their history has shown – also to people who did not know it – that in Mathematics there are still lots of open problems: even the investigation of polygons includes some questions that have not been solved yet in spite of being very old.
What does it mean to classify polygons? It means to determine a “sound” criterion to divide them in “classes” so that it is possible to say that two polygons in the same class are of the same type (or they are equal, if you prefer) and that two polygons in different classes are not of the same type (or they are different). Clearly, there is not only one way of distinguishing different types of polygons. Obviously, the choice of these different ways depends on the problem we are studying.

A first criterion, a bit rough, is to put two polygons in the same class if they have the same number of vertices. Doing so, all triangles are “equal”, all quadrilaterals are “equal”, but a quadrilateral and a triangle are different. In this way, we do not take into account the convexity or non-convexity of a polygon.
Then, we can try to refine the previous criterion to have just one triangle (all triangles are “equal”), only two quadrilaterals (one of them is convex and the other is concave), but four different types of pentagons (the convex, that with only a concave angle, that with two consecutive concave angles and that with two non-consecutive concave angles).
A criterion, which can be used to come up with this classification, requires that two polygons belong to the same class if it possible to “drag” the vertices of the first polygon onto the vertices of the second one (or of its mirror image) without falling into a middle “pathological” situation in which three vertices are aligned. (The existence of such a “catastrophic” situation, which is necessary to send a convex quadrilateral in a concave one, shows exactly that these two types of polygons are different).

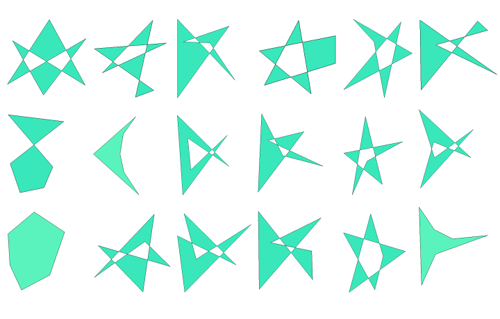
If you include the twisted polygons, the description is more interesting and articulate. It is known that there are (in addition to one type of triangle)
• 3 types of quadrilaterals (one of them is convex, one of them is concave and one of them is twisted)
• 11 types of pentagons (one of them is convex, three of them are concave, seven of them are twisted)
• 72 types of hexagons (one of them is convex, seven of them are concave and sixty-four are twisted).

It is not known if there exists a general formula that gives for any integer n the number of different types of n-gons according to this criterion.