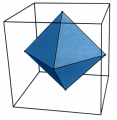
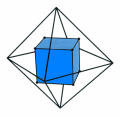
All of us agree on the fairness of using a cube, or indeed any of the regular polyhedra, to play dice, because any face has the same chance to appear. From a mathematical viewpoint, we say that the faces are indistinguishable. But what does "indistinguishable" mean exactly?

This adjective indicates that if we have a regular polyhedron and we choose two of its faces, we can always rotate it so that the first face goes to occupy the place of the second one and, as a whole, the polyhedron goes back onto itself.

For example, to move the front face of the cube in the figure to the position of the top face, it is enough to rotate the cube through 90 degrees about the straight line labelled r in the figure.

The same thing happens for two vertices or two edges:
to send edge a onto edge b we can rotate the cube through 120 degrees
about the straight line s; the same rotation can be used to send vertex P onto vertex Q.

The table below describes the five regular polyhedra.

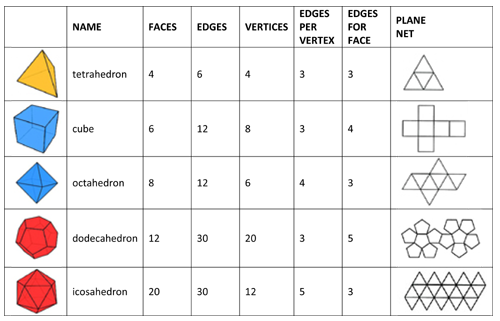
In the table, the first three columns of numbers give the number of faces,
edges, and vertices for each polyhedron; the two following columns can be interpreted as the "instructions" to build the skeleton of the polyhedron if sticks of the same length together with something to connect their ends are available (for example some drinking straws with pipe-cleaners for making joints). So the numbers that appear in the last row tell us that in an icosahedron there are 20 faces, 30 edges, 12 vertices, 5 edges meeting at each vertex, and 3 edges per face: if we join straws five at each vertex and form triangular faces, the object will "close itself" and give rise to an icosahedron.

The last column in the table also gives instructions for building the corresponding polyhedron: in this case, starting from the drawing of the net on cardboard we can obtain the surface of the polyhedron, rather than just its skeleton.

There is other information that we can extract from the table.
For example, the fact that the cube and the octahedron are "relatives":
they have the same number of edges, and each of them has as many faces as the other has vertices.
Also, the number of edges meeting at any vertex of one is the same as the number of edges around a face of the other.
This relationship is made clearer in the figures on the left: if we consider the centres of the faces of a cube and we join two of them when the corresponding faces have an edge in common, we will obtain an octahedron.

And vice versa, if we perform the same procedure starting from an octahedron, we will obtain a cube.

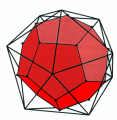
Also the dodecahedron and the icosahedron are relatives, in the sense above, as we can notice both from the numbers in the table and the figures.

Now what is "relative" of the tetrahedron?
There are no more regular polyhedra, but the fact that the regular tetrahedron has as many faces as vertices may help you to find an answer.