The orbifold shop and Conway’s “magic theorem”: the case of wallpaper groups
A nice interpretation (due to Conway) of the analysis of the different cases corresponding to wallpaper groups is the following: let’s imagine a
shop selling all the different components
we just described, that is the various parts which constitute an orbifold: conical points, corner points, mirror lines (corresponding to a star *), miracles (corresponding to a cross ×) and wonder-rings (corresponding to a dot
o).
Each one of these components has a cost and the price list is the following:
- a dot ο costs 2 Euros
- a cross × costs 1 Euro
- a star * costs 1 Euro
- a conical point n of order n costs (n-1)/n
- a corner point n of order n costs (n-1)/2n.
The “magic theorem” (so called by Conway) states then that a plane orbifold (corresponding to a wallpaper group) costs exactly 2 Euros, and that any combination of symbols costing exactly 2 Euros gives rise to a plane orbifold.
Then, we can discover with a bit of patience all the possible mixing of dots, crosses, stars, conical points and corner points giving a total cost of 2 Euros, and we find, as a corollary of the magic theorem, that there are exactly 17 possible cases; precisely they are:
The orbifold shop and Conway’s “magic theorem”: the case of friezes
Dealing with friezes, we have a new symbol (
∞) in the signature, costing 1 Euro if it appears before any star
*, and costing 50 cents if it appears after a star; this choice is consistent with the fact that the limit of
(n-1)/n, when n tends to infinity, is 1, while the limit of (n-1)/2n, when n tends to infinity, is 1/2. Thus, we can imagine it associated to a “center of infinite order”, corresponding now to a translation instead of a rotation.
The magic theorem for friezes asserts then that we get a plane orbifold corresponding to a frieze if and only if the total cost is exactly 2 Euros and the signature contains at least one symbol
∞.
Here also, with a bit of patience, one can list all the possibilities, and there are 7 of them:
The orbifold shop and Conway’s “magic theorem”: the case of finite groups of space isometries
We said that Conway’s notation works also for all possible symmetry types corresponding to finite groups of space isometries (and the quotients are the so called elliptic orbifolds).
In this case, when we speak of a quotient, we are referring to the quotient of a sphere (not of the whole 3d space) with respect to the group.
In order to understand where this sphere comes from, let’s begin with the example of a
cube (which is here the analogous of the drawing in the plane
case). The symmetry group of the cube consists of all space isometries sending the cube to itself. All these isometries fix the same point, the center of the cube; thus, they fix also any sphere with center in this point.
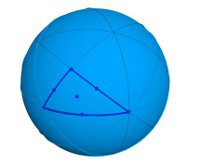
On the
sphere we see here (and that we should imagine with center in
the center of the cube) we see a slightly different colour to mark different parts corresponding to the faces of the cube (as if a blue cube, with faces a bit darker or lighter, had been blown up to a sphere); the lines we see are great circles on the sphere and they correspond to the intersection of the sphere with the (9) symmetry planes of the cube; the triangles are the projections on the sphere of the 48 (=6×8) triangles that we obtain on the cube if we cut it with its symmetry planes all together.
When we speak of an orbifold in a situation like the symmetry group of the cube, we refer just to the quotient of this sphere with respect to the group:
thus, in order to get the orbifold, we do on the sphere the same operations we did in the
first examples starting from the plane: that is, we have to identify all the points of the sphere which lie in the same orbit with respect to the symmetry group of the cube.
For example, a point where 4 great circles meet
corresponds to the center of a face in the cube, and all these points are “the same” (meaning that one of these points is enough to represent them all in the quotient), because, for any two faces in the cube, we may find an element of the symmetry group of the cube (fixing the cube and) sending one of the two faces into the other one.
Similarly, a point where 3 great circles meet corresponds to a vertex of the cube, and all the 8 vertices lie in the same orbit, thus giving one single point in the quotient; lastly, a point where two great circles meet corresponds to the midpoint of an edge of the cube, and all these points also are in the same orbit. The quotient is a spherical triangle (with angles 90°, 60° and 45°), and we can describe this orbifold, like we did for plane orbifolds, with the Conway signature
*432.
If we then calculate the total cost of this orbifold, we get 1 + 3/8 + 2/6 + 1/4 = 1 + 23/24 <2.
This is not a coincidence.
In fact, the magic theorem asserts in these cases that in order to get one of these orbifolds (which are named
elliptic orbifolds, and are the quotients of a sphere with respect to a finite group of isometries) it is necessary that the total cost is less than 2 Euros.
The different possibilities we get for building a Conway signature with total cost less than 2 Euros include 7 different cases which are:
but also some infinite families (depending on a natural number n):
For plane orbifolds, the total cost equal to 2 Euros was both a necessary and a sufficient condition for the existence of the corresponding orbifold.
Instead, for elliptic orbifolds, the situation is not exactly the same: in fact, there are 4 cases which have to be excluded because they do not give rise to an orbifold. They are:
| n | *n | nk(when n≠k) | *nk(when n≠k) |
So we are left with 7 infinite families:
Maybe some of you noticed the analogy between these 7 families and the 7 frieze groups; not only the numbers are the same and we get 7 possibilities in both situations, but, if we examine the list of the 7 Conway signatures in both cases, we realize that we can pass from one to the other one simply by substituting a
n” with a “
∞”:
Of course, this is not a coincidence and there are also some
decorations illustrating very well the reason of this analogy. Starting from a frieze, we can imagine to roll it up on a cylinder in such a way that the translation (which was sending any element to the “next” one) becomes now a rotation. Sometimes, we even give for granted, without realizing it, this analogy, for example when we very naturally call “frieze” a motif decorating (for example) the cylindrical
apsis of a church (thus surely not a planar decoration!); we see it as a frieze, simply because we automatically imagine the same decoration spread out on the facade.
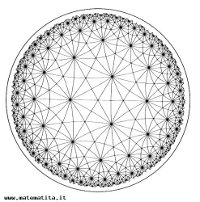
Completely different (and much more various and rich) is the situation corresponding to (hyperbolic) orbifolds with a total cost greater than 2 Euros; in this case we can’t list all the possibilities, because they are infinitely many:
here we see an example with Conway’s signature
*237 (which has in fact a total cost of 1 + 1/4 +2/6 + 6/14 = 1+85/84 ›2).
4/4