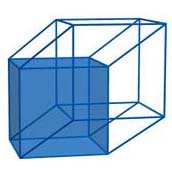
Who is the hypercube?
A net, a Schlegel diagram or a perspective projection: which of these three methods best represents a hypercube? The answer is: “ It depends”. In fact, they are three-dimensional representations of a hypercube, but they are not the hypercube. A representation may be more fit to study a property than the others.
For example, a Schlegel diagram seems to be the best for some combinatorial problems. On the other hand, it is more appropriate to choose a net for some optimization problems, such as finding the shortest path between two points. And yet, a projection shows more clearly that a hypercube contains infinitely many cubes. Having more than one representation allows one to get more information on the hypercube and its properties.

We “represent” a hypercube in three-dimensional space via a net, a Schlegel diagram or a projection. On the one hand, we loose the four-dimensional aspect of a hypercube; on the other hand, we have some representations which can be more easily manipulated and observed because they are three-dimensional.
In other words, we do not take into account the hypercube, but we study an (easier) picture of it from which we infer some properties of the hypercube.
Likewise, mathematicians have defined various “representations” of four-dimensional space, which are very diverse (algebraic, analytic….). They are more or less complicated and difficult to work with, but they allow mathematicians to observe and describe various characteristics of four-dimensional space.
As recalled before, a net, a Schlegel diagram and a projection are three-dimensional objects; however, it is worth mentioning that a net is the only one that differs from the other two because a Schlegel diagram is essentially a projection. If we identify the faces of a hypercube as required, we get a real hypercube. Actually, we can not do it in practice because our space is three-dimensional. It is a widely common technique in Topology to make up objects of higher dimension via identification of faces, segments, points, namely objects of smaller dimension. This technique allows one to make a great deal of diverse objects from simpler shapes.
For example, consider a square and identify the two pairs of opposite edges. If you assume the square be flexible and deformable, you can make these identifications in three-dimensional space: you will get a doughnut.
If you give a half-twist in one of the two identifications before you glue a pair of opposite edges (so as to change their direction), not only is the doughnut unobtainable, but it is actually not feasible to deform a square in three-dimensional space without forming any self-intersections. On the other hand, it is possible to make these identifications in four-dimensional space. This object is known as the Klein bottle.